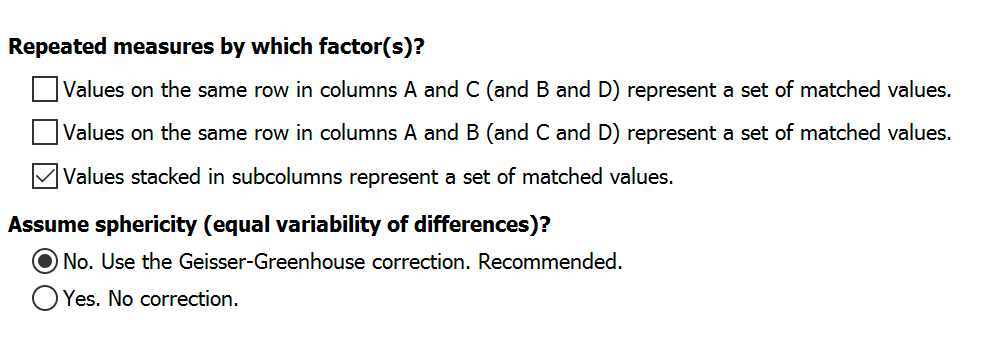
Repeated measures
Starting with Prism 8, three-way ANOVA can handle repeated measures. Three checkboxes let you specify which of the factors are, and are not, repeated measures. As you check and uncheck these options, look at the graphic on top of the screen which tries to show you the design you have selected.
Assume sphericity?
The concept of sphericity
The concept of sphericity is tricky to understand. Briefly it means that you waited long enough between treatments for any treatment effect to wash away.This concept is not relevant if your data are not repeated measures.
If you aren't sure, we recommend that you do not assume sphericity.
How your choice affects Prism's calculations
If you choose to not assume sphericity, Prism will:
•Include the Geisser-Greenhouse correction when computing the repeated measures ANOVA P value. The resulting P value will be higher than it would have been without that correction.
•Quantify violations of sphericity by reporting epsilon.
•Compute multiple comparisons tests differently.
If you ask Prism to assume sphericity, but in fact that assumption is violated, the P value from ANOVA will be too low. For that reason, if you are unsure whether or not to assume sphericity, we recommend that you check the option to not assume sphericity.
Random or fixed factors?
Read an advanced stats book to learn about random and fixed factors. Prism doesn't let you specify which factor(s) is/are random and which is/are fixed. It uses this rule:
•The row and column factors are considered to be fixed factors. You care about those exact levels of the factor. If there are three separate treatments, you care about those treatments. If there are four separate concentrations, you care about those concentrations (and have not selected those concentrations at random from all possible concentrations).
•When you choose repeated measures ANOVA, the participant or animal or subject is considered to be a random factor. You don't care about these particular participants or animals or whatever. Instead, they are assumed to be randomly selected from a larger population that you care about.