What is the law of mass action?
Analysis of radioligand binding experiments is based on a simple model, called the law of mass action. This model assumes that binding is reversible.

Binding occurs when ligand and receptor collide due to diffusion, and when the collision has the correct orientation and enough energy. The rate of association is:
Number of binding events per unit of time =[Ligand]⋅[Receptor]⋅kon.
Once binding has occurred, the ligand and receptor remain bound together for a random amount of time. The probability of dissociation is the same at every instant of time. The receptor doesn't "know" how long it has been bound to the ligand. The rate of dissociation is:
Number of dissociation events per unit time = [ligand×receptor]×koff.
After dissociation, the ligand and receptor are the same as at they were before binding. If either the ligand or receptor is chemically modified, then the binding does not follow the law of mass action.
Equilibrium is reached when the rate at which new ligand×receptor complexes are formed equals the rate at which the ligand×receptor complexes dissociate. At equilibrium:
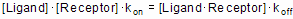
Meaning of Kd
Rearrange that equation to define the equilibrium dissociation constant Kd.
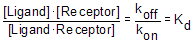
The Kd has a meaning that is easy to understand. Set [Ligand] equal to Kd in the equation above. The Kd terms cancel out, and you will see that [Receptor]/ [Ligand×Receptor]=1, so [Receptor] equals [Ligand×Receptor]. Since all the receptors are either free or bound to ligand, this means that half the receptors are free and half are bound to ligand. In other words, when the concentration of ligand equals the Kd, half the receptors will be occupied at equilibrium. If the receptors have a high affinity for the ligand, the Kd will be low, as it will take a low concentration of ligand to bind half the receptors.
The term "dissociation constant"
Don't mix up Kd, the equilibrium dissociation constant, with koff, the dissociation rate constant. They are not the same, and aren't even expressed in the same units.
Variable |
Name |
Units |
kon |
Association rate constant or on-rate constant |
M-1 min-1 |
koff |
Dissociation rate constant or off-rate constant |
min-1 |
Kd |
Equilibrium dissociation constant |
M |
Fractional occupancy
The law of mass action predicts the fractional receptor occupancy at equilibrium as a function of ligand concentration. Fractional occupancy is the fraction of all receptors that are bound to ligand.
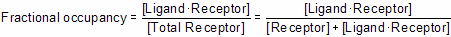
This equation is not useful, because you don't know the concentration of unoccupied receptor, [Receptor]. A bit of algebra creates a useful equation.

This equation assumes equilibrium. To make sense of it, think about a few different values for [Ligand].
[Ligand] |
Fractional Occupancy |
0 |
0% |
1.Kd |
50% |
4.Kd |
80% |
9.Kd |
90% |
99.Kd |
99% |
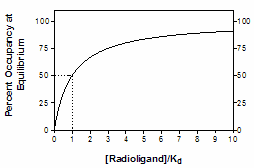
Note that when [Ligand]=Kd, fractional occupancy is 50%.
Assumptions
Although termed a "law", the law of mass action is simply a model that can be used to explain some experimental data. Because it is so simple, the model is not useful in all situations. The model assumes:
•All receptors are equally accessible to ligands.
•Receptors are either free or bound to ligand. It doesn't allow for more than one affinity state, or states of partial binding.
•Binding does not alter the ligand or receptor.
•Binding is reversible.
Despite its simplicity, the law of mass action has proven to be very useful in describing many aspects of receptor pharmacology and physiology.